Important RGPV Question

ME-502 MECHANICAL VIBRATION

V Sem, ME

UNIT 1- Fundamental Aspects of Vibrations

Q.1) What are main causes of vibrations?

(RGPV June 2023)

Q.2) A harmonic force F sinω acts on a displacement xo sin(x-3). If Fo=100, x = 0.02 m and w=2n rad/sec.
Find the work done during
i) First cycle
ii) The first second
iii) The first quarter second

(RGPV June 2023)

Q.3) Find the natural frequency of the car with following conditions: Total mass of car = 300 kg, wheel base 3.0 m centre of gravity of the car is 1.5 m from the front axle, radius of gyration is 1.0 m and spring constants of front and rear springs are 70×103 N/m each.

(RGPV June 2023)

Q.4) Find the natural frequency of torsional oscillations for the system shown in Fig.1. Take G=0.83×10¹¹ N/m² and neglect the inertia effect of the shaft.

(RGPV June 2023)

                                                                    

Q.5) In a spring mass vibrating system, the natural frequency of vibration is 3.56Hz. When the amount of the suspended mass is increased by 5kg, the natural frequency is lowered to 2.9 Hz. Determine the original unknown mass and the spring constant. 

(RGPV Nov 2022)

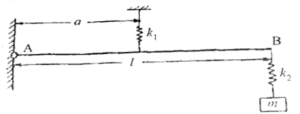
Q.6) A system is shown in the following figure 2. The bar AB is assumed to be rigid and weightless. The natural frequency of vibration of the system is given by

(RGPV Nov 2022)

                                                   

Q.7) Derive expression for two natural frequencies for small oscillations of the pendulum stain in figure 3 in the plane of the paper, assumes rods as massless and rigid Also obtain expressions for angular amplitude ratio in the two rods.

(RGPV June 2020)

                                                     

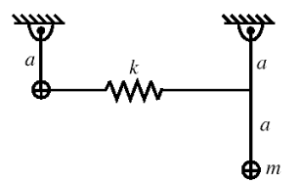
Q.8)  For the system shown in figure 2 below k₁ = 2000 N/m, k₂ = 1500 N/m, k₁ = 3000 N/m, k₁ = k = 500 N/m find m such that the system has a natural frequency of 10 Hz.          (RGPV June 2020)

                                                                         

 

Q.9) Derive the differential equation of the motion for a spring controlled simple pendulum shown in figurel below. The spring is in its unstretched position when the pendulum rod is vertical.  

(RGPV June 2020)

                                                       

Q.10) Define the following terms?
i) Vector representation of the displacement, velocity, and acceleration of SHM
ii) Fundamental mode of vibration
iii) Mathematical definition of Simple Harmonic Motion (SHM)

(RGPV Nov 2022)

Q.11) Enlist causes of Vibration? Advantages and disadvantages of Vibrations. 

(RGPV June 2020)

Q.12) A body describes simultaneously two motions:

x1= 3 sin 40 t, x2 = 4 sin 41 t.
What are the maximum and minimum amplitude of the combined motion and what is the best frequency?

(RGPV June 2020)

Q.13) Define degree of freedom of a system. Give examples of different types of degree of freedom system.

(RGPV June 2023)

UNIT 2-Damped Free Vibrations & Viscous damping

Q.1) What is viscous damping? Explain.

(RGPV June 2023)

Q.2) A damper offers resistance 0.05 N at constant velocity of 0.04 m/sec. The damper is used with k = 9 N/m. Determine the damping and frequency of the system when the mass of system is 0.1 kg.

(RGPV June 2023)

Q.3) The damped natural frequency of a system as obtained from a free vibration test is 10.5 Hz. During the forced vibration test with constant excitation force the maximum amplitude of vibration is found to be 9.5 Hz. Find the damping factor and it’s natural frequency.

(RGPV June 2023)

Q.4) A vibratory system consists of a mass 12.5 kg, a spring of stiffness 1000 N/m, and a dashpot with damping coefficient of 15 Ns/m. Find the value of critical damping of the system and the value of logarithmic decrement of the vibrating system.

(RGPV Nov 2022)

Q.5) Why we use Viscous damping for modelling the vibrating system. Explain in brief.

(RGPV Nov 2022)

Q.6) Derive the expression for frequency of free damped vibrations.

(RGPV Nov 2022)

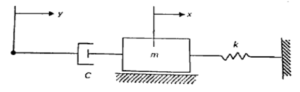
Q.7) Draw the FBD of the system and derived the differential equation governing by vibrating system is (Figure 1) 

(RGPV Nov 2022)

                                                   

UNIT 3- Harmonically excited Vibration

Q.1) Define critical speed of a system.

(RGPV June 2023)

Q.2) Prove that the critical speed of whirling speed for a rotating shaft is same as the frequency of natural transverse vibration.

(RGPV Nov 2022)

Q.3) What are the principles on which a Vibrometer and an accelerometer are based? Derive the expression.

(RGPV Nov 2022)

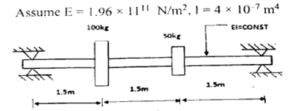
Q.4) A right cantilever steel shaft of 0.3 m effective length has a heavy rotor fixed at its end. The mass of the rotor is 10 kg and has a radius of gyration of 12 cm about its axis. The thickness of the rotor is 6 cm. The moment of inertia of the section of the shaft about its neutral axis is 10 cm². This shaft is to run at 10,000 rpm. Check if this operating speed is safe.

(RGPV Nov 2022)

Q.5) Determine the power required to vibrate a spring mass dashpot system with an amplitude of 1.5cm and at a frequency of 100Hz. The system has a damping factor of 0.05 and a damped natural frequency of 22 Hz as found out from the vibration record. The mass of the system is 0.5 kg.

(RGPV June 2020)

UNIT 4-Systems With Two Degrees of Freedom 

Q.1) A uniform rigid slender bar of mass 10 kg, hinged at the left end is suspended with the help of spring and damper arrangement as shown in the figure where K = 2 kN/m. C = 500 Ns/m and the stiffness of the torsional spring kΘ is 1 kN/m/rad. Ignore the hinge dimensions.
i) Find the un-damped natural frequency of oscillations of the bar about the hinge point.
ii) The damping coefficient in the vibration equation.

                                                 

(RGPV Nov 2022)

Q.2) Find the natural frequencies and first two normal mode shapes of the system shown in Figure 3.

(RGPV Nov 2022)

                                                

Q.3) Find the lowest natural frequency of vibration of system shown in Figure 4.

(RGPV Nov 2022)

                                                   

UNIT 5-Noise Engineering Subjective response of sound

Q.1) Discuss response of human to noise.

(RGPV June 2023)

Q.2) What precautions and remedies are adopted to avoid noise of machines?

(RGPV June 2023)

Q.3) Write the Logical Explanation with neat sketch if needed:
i) What is SPL in noise?
ii) Does 0db SPL mean no sound pressure?
iii) How loud is 100dB SPL?

(RGPV Nov 2022)

Q.4) What is octave band analysis of sound?

(RGPV Nov 2022)

Q.5) Write short notes on followings.
a) Sound pressure level and sound intensity scale
b) Main sources of noise on road and in industries
c) Strategies of noise control
d) Octane Band Analysis

(RGPV June 2020)

 EXTRA QUESTION-

Q.1) Write short notes on any two:
a) Energy method of solving vibration problem
b) Solid and structural damping
c) Dynamic vibration absorber
d) Measurement of noise

(RGPV June 2023)

— Best of Luck for Exam —